Statistical Significance Calculator
Introduction
In the world of statistics, understanding statistical significance is paramount. It helps researchers determine if the results of an experiment are likely to be due to chance or if they represent a true effect. However, calculating statistical significance manually can be complex and time-consuming. This is where a statistical significance calculator comes into play. In this article, we’ll delve into how to effectively use such a calculator, its underlying formula, provide an example solve, address frequently asked questions, and conclude with the importance of this tool in statistical analysis.
How to Use
Using a statistical significance calculator involves inputting relevant data from your experiment and specifying the desired confidence level. The calculator then processes this information to determine whether the observed differences are statistically significant or not.
To use the calculator:
- Input the necessary parameters such as sample sizes, means, and standard deviations.
- Specify the confidence level (usually set at 95%).
- Click the “Calculate” button to obtain the result.
Formula
The most commonly used formula for calculating statistical significance is based on the standard normal distribution, also known as the Z-test. It is given by:
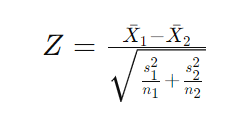
Where:
- Xˉ1 and Xˉ2 are the sample means of the two groups.
- s1 and s2 are the standard deviations of the two groups.
- n1 and n2 are the sample sizes of the two groups.
Example Solve
Let’s consider an example where we have two groups, A and B, with the following data:
- Group A: n1=50,Xˉ1=25,s1=5
- Group B: n2=50,Xˉ2=30,s2=6
Assuming a 95% confidence level, we can calculate the Z-value using the formula mentioned earlier:

After calculating, we find that Z=−2.474.
FAQs
Q: What is statistical significance?
A: Statistical significance indicates whether the observed differences between groups or variables are likely to be real or due to chance.
Q: Why is statistical significance important?
A: It helps researchers make informed decisions based on data analysis and ensures the reliability of study results.
Q: What is the significance of the confidence level?
A: The confidence level represents the probability that the calculated confidence interval includes the true population parameter.
Q: Can statistical significance guarantee the practical importance of results?
A: No, statistical significance only indicates the reliability of results, not their practical significance or importance.
Conclusion
In conclusion, a statistical significance calculator simplifies the process of determining the significance of experimental results. By understanding how to use it effectively, researchers can make informed decisions based on solid statistical analysis, ensuring the credibility and reliability of their findings.